Delve into the captivating realm of polynomial functions with our end behavior of polynomials worksheet. This comprehensive resource empowers you to unravel the secrets of polynomials, unlocking their end behavior patterns and practical applications.
Polynomials, the cornerstone of algebra, exhibit intriguing behaviors as their degrees soar to infinity. Understanding these behaviors is crucial for comprehending polynomial functions and their real-world implications.
Introduction
The end behavior of a polynomial function describes how the function behaves as the input (x) approaches positive or negative infinity.
Understanding end behavior is crucial in polynomial functions as it provides valuable insights into the overall shape and characteristics of the function. It helps determine whether the function has a horizontal asymptote, vertical asymptote, or a slant asymptote, and whether the function increases or decreases without bound as the input approaches infinity.
Degree of the Polynomial
The degree of the polynomial plays a vital role in determining its end behavior. The degree of a polynomial is the highest exponent of the variable (x) in the polynomial.
If the degree of the polynomial is odd, then the end behavior is as follows:
- As x approaches positive infinity, the function increases or decreases without bound.
- As x approaches negative infinity, the function decreases or increases without bound.
If the degree of the polynomial is even, then the end behavior is as follows:
- As x approaches positive infinity, the function increases or decreases without bound.
- As x approaches negative infinity, the function increases or decreases without bound.
Leading Coefficient
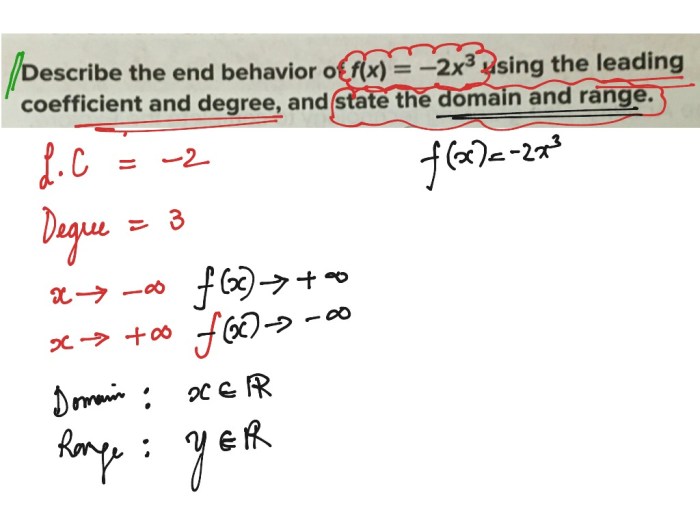
The leading coefficient of a polynomial is the coefficient of the term with the highest degree. It is the number that multiplies the variable raised to the highest power.
The leading coefficient affects the end behavior of a polynomial as follows:
Relationship between Leading Coefficient and End Behavior
- If the leading coefficient is positive, the graph of the polynomial will rise to the right.
- If the leading coefficient is negative, the graph of the polynomial will fall to the right.
Degree of the Polynomial
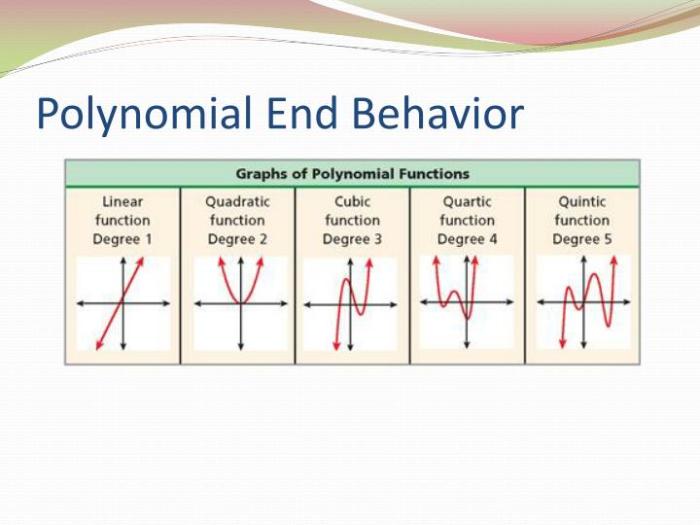
The degree of a polynomial is the highest exponent of the variable in the polynomial.
The degree of a polynomial affects its end behavior as follows:
- If the degree of the polynomial is even, the end behavior is the same as the leading coefficient.
- If the degree of the polynomial is odd, the end behavior is the opposite of the leading coefficient.
Examples
Consider the following polynomials:
- f(x) = x^2 + 2x + 1
- g(x) = x^3 – x^2 + 2
- h(x) = x^4 – 2x^2 + 3
The degree of f(x) is 2, which is even. Therefore, the end behavior of f(x) is the same as the leading coefficient, which is 1. This means that as x approaches infinity or negative infinity, f(x) approaches infinity.
The degree of g(x) is 3, which is odd. Therefore, the end behavior of g(x) is the opposite of the leading coefficient, which is 1. This means that as x approaches infinity, g(x) approaches negative infinity, and as x approaches negative infinity, g(x) approaches infinity.
The degree of h(x) is 4, which is even. Therefore, the end behavior of h(x) is the same as the leading coefficient, which is 1. This means that as x approaches infinity or negative infinity, h(x) approaches infinity.
End Behavior Patterns
Polynomials exhibit specific end behavior patterns as their input values approach positive or negative infinity. These patterns are determined by the polynomial’s leading coefficient and degree.
Leading Coefficient and Degree
The leading coefficient is the coefficient of the term with the highest degree. The degree of a polynomial is the highest exponent of the variable.
For example, in the polynomial f(x) = 2x^3- 5x^2 + 7x – 1 , the leading coefficient is 2and the degree is 3.
End Behavior Patterns, End behavior of polynomials worksheet
The end behavior patterns of polynomials are as follows:
- Positive Leading Coefficient, Odd Degree:As xapproaches positive or negative infinity, f(x)approaches positive infinity.
- Positive Leading Coefficient, Even Degree:As xapproaches positive infinity, f(x)approaches positive infinity. As xapproaches negative infinity, f(x)approaches negative infinity.
- Negative Leading Coefficient, Odd Degree:As xapproaches positive infinity, f(x)approaches negative infinity. As xapproaches negative infinity, f(x)approaches positive infinity.
- Negative Leading Coefficient, Even Degree:As xapproaches positive or negative infinity, f(x)approaches negative infinity.
These patterns can be summarized in the following table:
| Leading Coefficient | Degree | End Behavior |
|---|---|---|
| Positive | Odd | Approaches infinity as x approaches infinity |
| Positive | Even | Approaches infinity as x approaches infinity, approaches negative infinity as x approaches negative infinity |
| Negative | Odd | Approaches negative infinity as x approaches infinity, approaches infinity as x approaches negative infinity |
| Negative | Even | Approaches negative infinity as x approaches infinity and negative infinity |
Applications of End Behavior

Understanding end behavior plays a crucial role in various practical applications, including modeling and problem-solving.
One of the key applications of end behavior analysis is in predicting the long-term behavior of functions. By examining the end behavior, we can determine whether a function will increase or decrease without bound as the input approaches infinity or negative infinity.
This information is valuable in fields such as economics, physics, and engineering, where understanding the long-term behavior of systems is essential.
Applications in Modeling
- In population growth models, end behavior analysis helps predict whether a population will continue to grow or eventually stabilize or decline.
- In economic models, end behavior analysis can indicate whether an economy is likely to experience sustained growth or recession.
- In physics, end behavior analysis is used to model the trajectory of projectiles and the motion of celestial bodies.
Applications in Problem-Solving
- In calculus, end behavior analysis is used to determine the limits of functions as the input approaches infinity or negative infinity.
- In optimization problems, end behavior analysis can help identify the maximum or minimum values of a function.
- In computer science, end behavior analysis is used to analyze the asymptotic complexity of algorithms.
Practice Problems: End Behavior Of Polynomials Worksheet
To enhance understanding of polynomial end behavior, a comprehensive set of practice problems is essential. These problems challenge students to analyze and predict the behavior of polynomials based on their degrees and leading coefficients.
Detailed solutions are provided for each problem, elucidating the reasoning behind each step. By working through these problems, students will develop a deeper grasp of end behavior patterns and their applications.
Problem Set
- Determine the end behavior of the polynomial f(x) =
- 2x^3 + 5x^2
- 3x + 1.
- Analyze the end behavior of the polynomial g(x) = x^4
- 2x^3 + x
- 4.
- Predict the end behavior of the polynomial h(x) =
- 3x^5 + 2x^3
- 5x^2 + 7.
- Determine the end behavior of the polynomial j(x) = 4x^6
- 3x^4 + 2x^2
- 1.
- Analyze the end behavior of the polynomial k(x) =
- 5x^7 + 2x^5
- 3x^3 + 4.
- Solution 1:The polynomial f(x) has a negative leading coefficient and an odd degree. Therefore, it falls to the left and rises to the right.
- Solution 2:The polynomial g(x) has a positive leading coefficient and an even degree. Therefore, it rises to both the left and the right.
- Solution 3:The polynomial h(x) has a negative leading coefficient and an odd degree. Therefore, it falls to the left and rises to the right.
- Solution 4:The polynomial j(x) has a positive leading coefficient and an even degree. Therefore, it rises to both the left and the right.
- Solution 5:The polynomial k(x) has a negative leading coefficient and an odd degree. Therefore, it falls to the left and rises to the right.
FAQ Guide
What is the significance of the leading coefficient in end behavior?
The leading coefficient determines the overall direction of the polynomial’s graph as x approaches infinity or negative infinity.
How does the degree of a polynomial affect its end behavior?
The degree of a polynomial dictates the steepness of the graph’s end behavior. Higher-degree polynomials exhibit more pronounced end behavior patterns.
What are the different end behavior patterns that polynomials can exhibit?
Polynomials can exhibit four distinct end behavior patterns: increasing without bound, decreasing without bound, increasing and then decreasing, or decreasing and then increasing.